Numerical Methods for PDEs and Integral Equations

Dr. Akash Anand, Department of Mathematics & Statistics

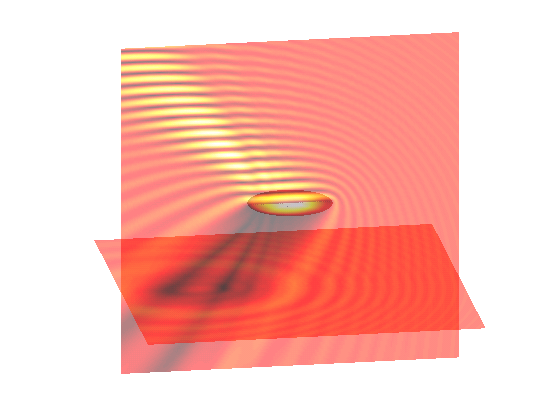
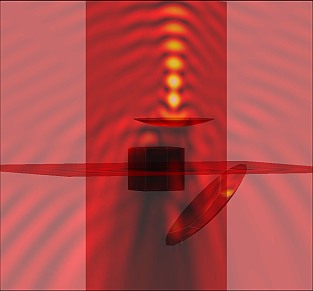
My primary research interests are in the area of numerical methods for partial differential equations and integral equations. More specifically, I specialize in high order efficient numerical schemes for solutions of such equations. In particular, my research work belongs to the field of computational electromagnetics and acoustics and deals with efficient high-order integral equation methods for surface and volumetric scattering in two and three dimensions. The main goal of computational electromagnetics and acoustics is to design and implement numerical schemes that can be used to efficiently simulate electromagnetic and acoustic wave interactions with complex material structures. Encompassing numerical methods for wave propagation and scattering, inverse problems and geometrical optics, computational electromagnetics and acoustics represent a broad and important area of present day computational science. Its spectacular growth in the past decades, largely enabled by concomitant developments in the computer industry, was primarily triggered by the increasing spectrum of its applications. Indeed, today applications are found in communications (transmission through optical fiber lines or wireless communication), remote sensing and surveillance (radar and sonar systems), geophysical prospecting, materials science and biomedical imaging (optical coherence tomography), to name a few.

Keywords: Numerical analysis, Scientific Computing, Integral Equations, Computational Acoustics and Electromagnetics